Student's t-Test (t₀, t_e & H₀) Calculator

Student's t-test calculator for test of significance (hypothesis) for single mean, difference between two means & two equal sample sizes (paired t-test) by using t-statistic (t₀) & critical value of t (t_e) for small samples of population in statistical surveys & experiments. This calculator is featured to generate the complete work for test of significance for small samples using one or two tailed t-test may helpful for grade school students to solve t-test worksheet or homework problems.

t-Test & it's Applications

Statistics & probability functions are used in almost every fields such as math, science, engineering, finance, health, digital networks, data processing, social concerns, etc to research & analyze the data distributions to draw conclusions to improve the quality of process or services. Regardless of any fields, user can analyze, model, design & test any statistical surveys or experiments by using these below calculators to get the summary of data.

Student's t-Test Formulas

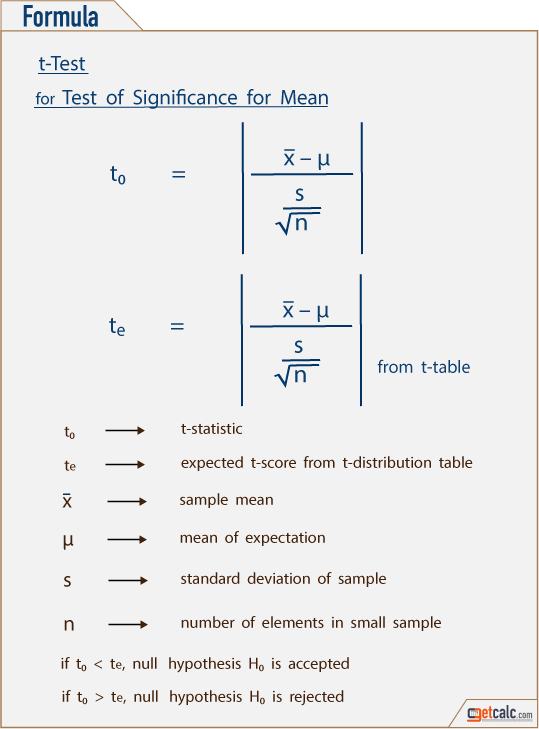
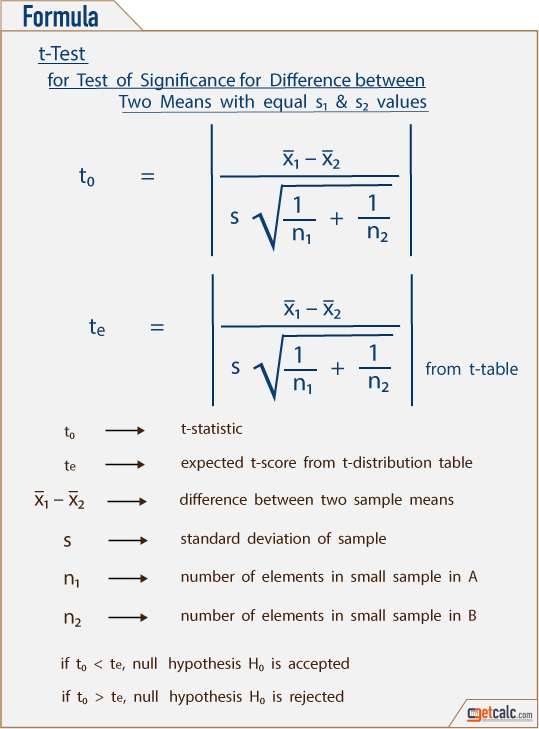
In the theory of statistics & probability, the below formulas are the mathematical representation to estimate the t-statistic for the test of significance for sample mean with unknown population variance, difference between two sample means with equal but unknown population variance & related samples with unknown population variance. Users may refer these below formulas to know what are all the parameters are being used in the test of hypothesis for small samples. Users may use this one or two tailed t-test calculator to verify the estimation along with the complete work for test of significance using t-distribution table.

t-test formula for test of hypothesis for sample mean

t-test formula for test of hypothesis for difference between two sample means

Critical Value (Rejected Region) from T-Distribution Table

In student's t-test, the t-distribution table is used to find the critical value of t_e at a stated level of significance such as 0.10, 0.50, 0.90, 0.99 level. For example, 1%, 5% & 25% significance represented by t_0.01, t_0.05 and t_0.25. This expected of t-value or t-critical t_e is compared with calculated or t-statistic t₀ in the statistical experiments to accept or reject the hypothesis H₀. The critical value of 5% for two tailed test lies on both sides of the distribution with the rejection area of t_ν = t_0.025 on each sides. In other words, t_ν at the significance level of 0.20 for single tailed t-test is equal to t_ν at the significance level of 0.10 on both sides. The critical value or rejection region of t-distribution for small samples can be obtained by referring the t-distribution table at stated level of significance such as 1%, 5%, 9%, 10% or 50% etc or 0.10, 0.50, 0.90, 0.99 etc.

Test of Significance using t-Test

The difference between the sample mean or means is not significant, if the calculated or t-statistic t₀ value is lesser than the rejection region value (t-critical) from the t-table. Therefore, the null hypothesis H₀ is accepted. The difference between the sample mean or means is significant, if the calculated or t-statistic t₀ value is greater than the rejection region (t-critical) value from the t-table. Therefore, the null hypothesis H₀ is rejected.

Solved Example Calculations

The below are the solved examples with step by step estimation for the test of significance for sample mean with unknown variance, test of significance for difference between two sample means with equal but unknown population variance & test of significance for relative samples (paired t-test) with unknown population variance using t-test. In addition to the below examples, users can generate complete work for one or two tailed t-test for any corresponding input values.

Solved Examples - Work with Steps

Student's t-Test (t0, te & H0) Calculator