Standard Deviation Calculator
Standard deviation calculator to estimate the degree of uncertainty or linear variability by using samples rather than the whole population dataset in statistical experiments. The degree of uncertainty shows how the sample population deviates from the mean or how the sample population is distributed around the mean. If you are involved into research or analysis like clinical trials, impact analysis, material strengths, manufacturing, sales trend, polling trend, chemical, mechanical, internet networks, digital computations, database, production etc., this standard deviation calculator is the best tool to analyze the data dispersion or study about the potentially larger population set by only using the smaller amount of data (sample population).
Standard deviation calculator not just plots your data and shows how the data is spread around the mean, but also provides the complete work with steps for each analysis you are doing with this online tool. Since the work with steps is available, as a researcher you can get to know what is the formula behind it and how all the parameters and dataset values are being used in the formula. This standard deviation calculator would be the best tool for early stage researchers to thoroughly understand the degree of uncertainty analysis. Besides, the examples of standard deviation real world problems definitely help users to understand in what context this tool can be used to find the uncertainty of events. Get started today with this standard deviation calculator to study about the large set of data by only using a smaller amount of relevant data. Finding uncertainty helps you to improve your quality of service or products.
Standard Deviation
Standard Deviation is a method used in the context of probability and statistics to find the degree of uncertainty or linear variability of data distribution from its mean (μ). In other words, it defines how the behavioral characteristics of the sample dataset of a larger data population deviates from its common behavioral characteristics. The mean is the common behavioral characteristics of the data distribution whereas the standard deviation is the square root of variance and plays an important role to characterize the data distribution around the mean to take the scientific assessment in the event of uncertainty and variation. It's a most important and widely used function to measure the dispersion. This behavioral characteristics deviation of the samples can be easily calculated by using this standard deviation calculator.
Standard Deviation Calculator: How to Identify the Data Deviation?
In the frequency distribution there would be the difference among the elements. By using a standard deviation calculator, you can identify how the elements of frequency distribution are spread around the mean.
- The lower dispersion indicates that there is a relatively large amount of elements uniformly distributed around the mean.
- The higher dispersion indicates that there is relatively less amount of elements uniformly distributed around the mean.
Standard deviation employs the complete dataset or sample of an infinite or potentially larger set of data. The statistical method used for finding the dispersion for a finite set of data is called as population standard deviation, often denoted by the Greek symbol σ. It is generally used in descriptive statistics which deals with a finite number of population elements. Whereas, the statistical method used to estimate the degree of uncertainty from the sample of larger or infinite data population is called sample standard deviation. It is often denoted by an English alphabet σ.
The most frequently used method in inferential statistics is sample standard deviation which deals with an infinite population dataset to draw the conclusion about the population in the statistical experiments. For sound estimation in statistical experiments, one must understand how to choose samples or how much amount of samples are required to make the experiments more reliable. To understand and find the right amount of samples from the larger population, use this sample size calculator.
Users may use this standard deviation calculator to find how the whole data is distributed around the mean or the spread of data distribution around the central location. Any business may analyze its quality of service or process by using this uncertainty calculation. The summary of data which describes the deviation from the expected results indicates how quality your process or service is. The method which deals with the finite number of population is called Descriptive Statistics and the method which deals with the infinite number of population is called Inferential Statistics.
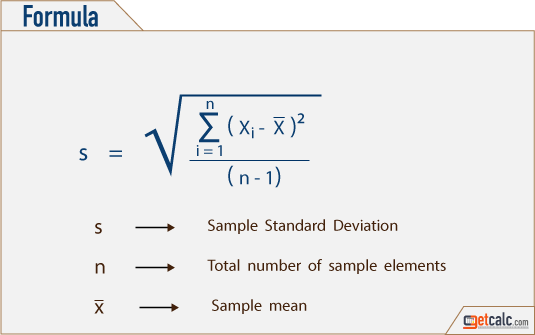
Formula: Standard Deviation
The below is the sample standard deviation formula to estimate the degree of uncertainty of the sample of an infinite or potentially larger dataset or population. The same formula is being used in this standard deviation calculator.
Standard Deviation Calculator for Real World Problems
Standard deviation is applicable in industries like scientific, engineering, finance, manufacturing, chemical, mechanical, manufacturing, internet networks, digital computations, database, production etc. to find the dataset characteristic deviation (degree of uncertainty) from the common characteristics of the dataset. It's a repeated analysis based on experiments with infinite or larger quantities of data to summarize the degree of dispersion in order to analyze the quality of service or process. The below are the fewer real world problems where the sample standard deviation calculator can be effectively used to analyze the characteristics of the sample dataset to draw the conclusions about the infinite or larger dataset population.
Use of Standard Deviation Calculator in Manufacturing
The below example is one of a standard deviation real world problems in manufacturing help early stage analysts and researchers to easily understand how to employ standard deviation in the manufacturing industry. For example, a car manufacturing company is making 15 inch diameter pistons for four stroke engines. The company randomly picked 10 pistons. The diameter of 10 pistons are 15, 15, 14.99, 15.01, 15, 15, 15.05, 15, 14.98 and 15 inches. What is the degree of uncertainty in the samples? What should be taken from the answer of standard deviation to improve the quality of process?
The characteristics of the samples deviates from the mean by 0.0171σ. The necessary changes are required in the manufacturing process to minimize the deviation as 0 to improve the quality of the product. Any manufacturing entity may use this standard deviation calculator to find the degree of uncertainty and improve the quality of process.
Use of Standard Deviation Calculator in Material Science
The analysis of material is an essential part for manufacturing industries. The standard deviation is used to analyze various materials to estimate the uncertainties while analyzing how strong the material is. In material design and analysis the sample standard deviation calculator can be effectively used to measure the linear variability of material strength. The below example is one of the standard deviation real world problems in material science, and may be helpful for engineers to understand how to analyze the strength of material by sampling.
For example, an entity is manufacturing connecting rods involving standard deviation to measure the linear variability of outcome or the possibility of defective outcomes. To do this, the experiment requires the molecules samples randomly collected at various points of the connecting rod. Let's say, a 15 inches connecting rod is involved in the experiment and the samples are collected at 1 inch, 2 inches, 2.5 inches, 3 inches, 5 inches, 7 inches, 7.5 inches, 13 inches, 14 inches and 14.3 inches length. How to find the degree of uncertainty?
From the results, what should be taken to improve the quality of the process? The whole samples' molecules present within the standard deviation of 1.9579σ. Since the standard deviation is not more than 2 or 3σ, therefore the variation in the sample elements is within the range and is acceptable. The summary of data says that the molecules of the connecting rod are almost equally distributed across its length. However, the corrective measures to be taken to bring the standard deviation as close to zero to improve the quality of process to have the molecules equally distributed across the rod so as to ensure the strength of material is equal at all points of the material. Using the increased number of sample points in the statistical experiments would maximize reliability of outcome. Using this standard deviation calculator helps you to quickly summarize the data and the degree of uncertainty from mean.

