Confidence Interval for Proportion of Infinite Population for n = 80, p = 0.30 & 90% confidence
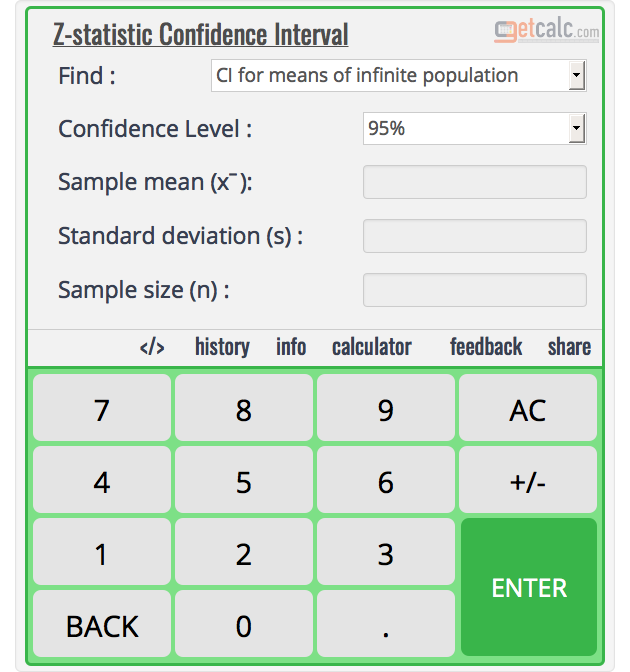The probability of unknown parameter of population expected to lie between the confidence interval 0.2158 and 0.3842. The values 0.2158 and 0.3842 are the lower and upper limits of the confidence interval for proportion of infinite population estimated from sample size n = 80, proportion of successes p = 0.30, & Z-score of confidence level for 90% = 1.645 to estimate the confidence interval for proportion of infinite population.Work with Steps for n = 80, p = 0.30 & 90% confidence
The complete work with step by step calculation for sample size n = 80, proportion of successes p = 0.30, & Z-score of confidence level for 90% = 1.645 to estimate the confidence interval for proportion of infinite population in statistical experiments.
Workout :
step 1 Address the formula input parameters and values
Sample size n = 80
Proportion = 0.30
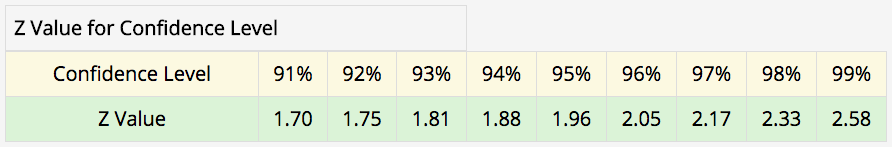
Z-score for 90% confidence level = 1.645
step 2 Substitute sample size, proportion of success & Z-score in the below confidence interval formula
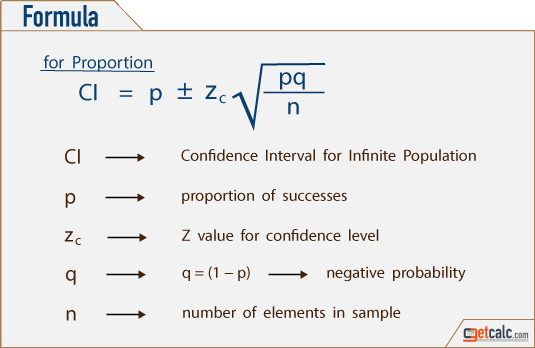
= 0.30 ± 1.645√(0.30 x 0.7)/80
= 0.30 ± 1.645√(0.21)/80
= 0.30 ± 1.645√0.002625
step 3 Simplify the above expression
= 0.30 ± 1.645 x 0.0512
= 0.30 ± 0.0842
0.30 + 0.0842 = 0.3842 or 0.30 - 0.0842 = 0.2158
The lower limit is 0.2158 and
The upper limit is 0.3842
0.2158 to 0.3842 is the confidence limits for n = 80, p = 0.30, & Z = 1.645
