Chi-squared Test (χ²0, χ²e & H0) Calculator
getcalc.com's Chi-squared (χ²) Test calculator is an online statistics & probability tool to estimate χ²-statistic (χ²0), χ²-critical value (χ²e) & test of hypothesis (H0) to check if the test of significance between two nonuniform datasets is accepted or rejected under null hypothesis in statistics & probability experiments.
Why χ²-Test & Formula
Chi-squared (χ2) Test is a technique used in probability & statistics to check if the results of χ²-distribution is statistically significant under null hypothesis. χ²-distribution is a collection of data which is not uniformly distributed over the period of time. Chi-squared test requires χ²-statistic χ²0 & critical (table) value of χ²-distribution χ²e at a stated level of significance (α = 1%, 2%, 3%, 4%, 5%, 10%, 25%, 5% etc or α = 0.01, 0.02, 0.03, 0.04, 0.05, 0.1, 0.25, 0.5 etc) for the test of hypothesis (H0) in statistics & probability surveys or experiments to analyze samples which is not distributed uniformly or normally. Critical value of χ2 from χ2-distribution table represents the rejection area of distribution. The estimated value of χ² or chi-squared statistic (χ²0) is compared with the critical value from χ²-distribution p-value table at a stated level of significance to check if the test of null hypothesis accepted in statistical experiments. Users may use this below χ²-test calculator to estimate χ²-statistic (χ²0), critical value (χ²e) & hypothesis test (H0) to test the significance between two or more samples which are not normally or uniformly distributed over time.
Inference
The below statements show when to accept or reject null hypothesis H0 in χ²-test
For null hypothesis H0 :
If F0 < Fe then the null hypothesis H0 is accepted.
It states that there is no significance difference between χ²-statistic & expected (critical) value of χ².
If χ²0 > χ²e then the null hypothesis H0 is rejected.
It states that there is significance difference between χ²-statistic & expected or critical value of χ².
The below is the mathematical representation for χ²-test formula to estimate the quality of variances among two or more samples which are not normally or uniformly distributed over time to predict the characteristics of population parameters of a unknown distribution.
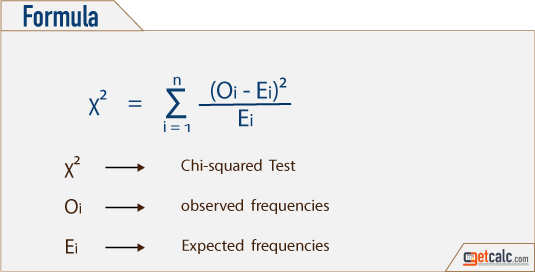
Solved Example Problems with Solutions
The below are the solved example problems for χ²-Test with step by step solution to analyze the two set of irregularly (nonuniform) distributed samples. Users may refer the below estimations to know what formula & input parameters are being used in the respective chi-squared calculations.

