Standard Error of Mean for n = 6 & Population 2, 3, 2.5, 4, 2.7, . . . . , 2.8 & 3.5
Calculation summary & work with steps for n = 6 & population 2, 3, 2.5, 4, 2.7, . . . . , 2.8 and 3.5 to estimate the standard error of sample mean. The below is the calculation summary for SE of x̄ for sample size n = 6 using population standard deviation σ = 0.6198.
| Calculation Summary | |
|---|---|
| Population | 2, 3, 2.5, 4, 2.7, . . . . , 2.8 & 3.5 |
| Sample size (n) | 6 |
| Population standard deviation (σ) | 0.6198 |
| Standard Error of mean (x̄) | 0.253 |
SE of x̄ Work with Steps for n = 6 & σ = 0.6198
The below is the example work with step by step calculation shows how to estimate the standard deviation of sampling distribution of mean for sample size n = 6 and population data set 2, 3, 2.5, 4, 2.7, 3.2, 4.1, 2.9, 2.8 and 3.5 to help grade school students to solve the similar SE of x̄ worksheet problems efficiently.
Workout :
step 1 Address the formula, input parameters & values
Input parameters & values
x1 = 2, x2 = 3, x3 = 2.5, . . . . , x10 = 3.5
Number of elements n = 10
Sample size = 6
Find SE of mean for 2, 3, 2.5, 4, 2.7, 3.2, 4.1, 2.9, 2.8 & 3.5
step 2 Find the mean for 2, 3, 2.5, 4, 2.7, 3.2, 4.1, 2.9, 2.8 & 3.5
µ =
n
∑
i = 1
Xin
=(2 + 3 + 2.5 + . . . . + 3.5)/10
µ = 3.07
step 3 Find the population standard deviation for 2, 3, 2.5, 4, 2.7, 3.2, 4.1, 2.9, 2.8 & 3.5
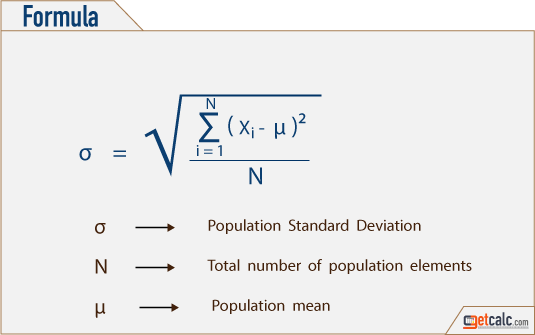
=√{ (2 - 3.07)² + (3 - 3.07)² + (2.5 - 3.07)² + . . . . + (3.5 - 3.07)²}/10
= √(-1.07)² + (-0.07)² + (-0.57)² + . . . . + (0.43)²/10
= √(1.1449 + 0.0049 + 0.3249 + . . . . + 0.1849)/10
= √3.841/10
= √0.3841
σ = 0.6198
step 4 Apply standard deviation and sample size values in the below standard error formula
SEμ = σ√n
=0.6198/ √6
= 0.253
For the dataset 2, 3, 2.5, 4, 2.7, 3.2, 4.1, 2.9, 2.8 & 3.5
3.07 is the sample mean
0.6198 is the population standard deviation
0.253 is the standard error of mean
