Normal Distribution Example for μ = 9, σ = 2.5, x1 = 3 & x2 = 3.5
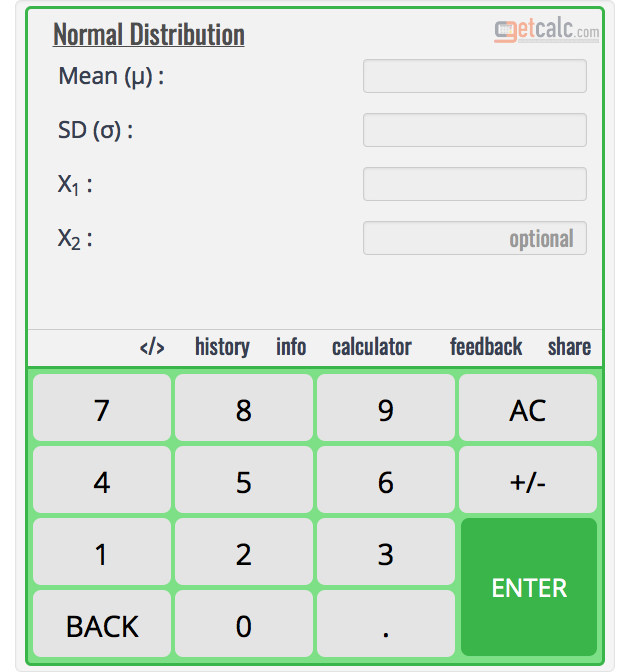
The below summary & example workout with step by step calculation shows how to estimate P(X < X1), P(X > X1), P(X < X2), P(X > X2) or P(X1 < X < X2) of normal (Gaussian) probability distribution for the input values of μ = 9, σ = 2.5, x1 = 3 & x2 = 3.5 may helpful for beginners to learn how the input parameters are being used in the Normal probability distribution formula in statistical experiments or useful for grade school students to solve such similar worksheet or homework problems efficiently.
| Calculation Summary | |
|---|---|
| mean (μ) | 9 |
| standard deviation (σ) | 2.5 |
| x1 | 3 |
| x2 | 3.5 |
| P(x < 3) | 0.009 |
| P(x > 3) | 0.991 |
| P(x < 3.5) | 0.0142 |
| P(x > 3.5) | 0.9858 |
| P(3 < x < 3.5) | 0.0052 |
Example Calculation for μ = 9, σ = 2.5, x1 = 3, x2 = 3.5
This below solved example for normal probability distribution with step by step calculation shows how the input parameters μ = 9, σ = 2.5, x1 = 3 & x2 = 3.5 are being used in the Gaussian distribution formula to find the probability of success P(X < X1), P(X > X1), P(X < X2), P(X > X2) or P(X1 < X < X2) in statistical experiments.
step 1Address the formula, input parameters and values
Mean (μ) = 9
Standard deviation (σ) = 2.5
x1 = 3
x2 = 3.5
step 2 Substitute mean, standard deviation, x1 & x2 value in the below normal distribution formula
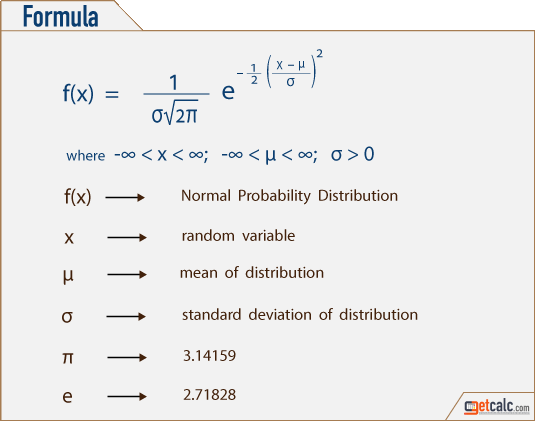
f(x1) = 12.5 x √ 2 x 3.14159e - (3 - 9)2 / [2 x (2.5)2]
= 12.5 x √ 2 x 3.14159e - (3.5 - 9)2 / [2 x (2.5)2]
step 3 Find P(x1)
= 12.5 x √ 2 x 3.14159e - (-6)2 / (2 x 6.25)
= 12.5 x √ 2 x 3.14159e - (36 / 12.5)
= 12.5 x √ 2 x 3.14159e - 2.88
= 12.5 x √ 2 x 3.14159 x 0.0561
= 12.5 x √6.2832 x 0.0561
= 12.5 x 2.5066 x 0.0561
= 16.2666 x 0.0561
= 0.1596 x 0.0561
P(x1) = 0.009
step 4 Find P(x2)
= 12.5 x √ 2 x 3.14159e - (-5.5)2 / (2 x 6.25)
= 12.5 x √ 2 x 3.14159e - (30.25 / 12.5)
= 12.5 x √ 2 x 3.14159e - 2.42
= 12.5 x √ 2 x 3.14159 x 0.0889
= 12.5 x √6.2832 x 0.0889
= 12.5 x 2.5066 x 0.0889
= 16.2666 x 0.0889
= 0.1596 x 0.0889
P(x2) = 0.0142
step 5 P(x2) to find P(x < 3), P(x > 3), P(x < 3.5), P(x > 3.5), P(3< X < 3.5) by using P(x1)
P(x < 3) = 0.009
P(x > 3) = 1 - P(x1)
P(x > 3) = 0.991
P(x < 3.5) = 0.0142
P(x > 3.5) = 1 - P(x2)
P(x > 3.5) = 0.9858
P(3 < X < 3.5) = 0.0052
