Binomial Distribution Calculator
getcalc.com's Binomial distribution calculator is an online statistics & probability tool to estimate the total combinations (nCr), probability of x number of successes P(x), mean (μ), variance (σ²) & standard deviation (σ), coefficient of skewness & coefficient of kurtosis from the n number of finite & repeated independent trials in statistical experiments. This calculator is featured to generate the complete work with steps for any corresponding input values to estimate P(x), μ, σ2, Skewness & Kurtosis.
Binomial Distribution & its Application
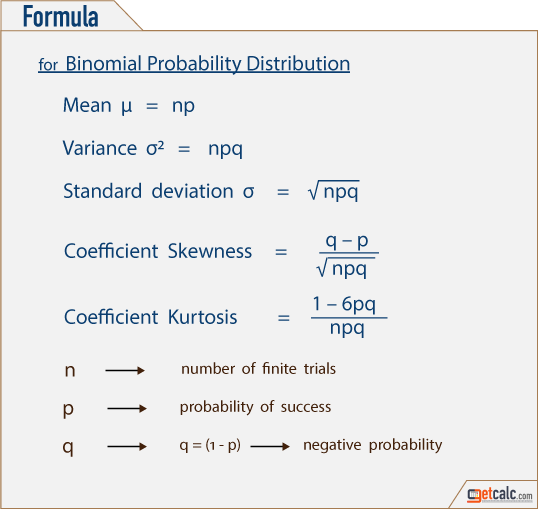
In the context of probability & statistics, it is said to be Binomial Distribution if the distribution has n number of finite & independent trials and the probability of success is constant for each trial only results in success or failure. It represents the probability of x number of successes in the n number of independent trials. Tossing a coin (head or tail), throwing a dice (odd or even) or drawing a card from deck of cards with replacement are some of the familiar examples of Binomial distribution. In the experiments, generally the success probability for each trial is taken in to account to find the probability for x number of successes in the series of n events. The product of probability of success and n number of trials is the mean, the product of mean & the failure probability is the variance, the square root of variance is the standard deviation, the ratio of difference between negative & success probability to standard deviation is the coefficient of skewness and the ratio between 6 times of success & failure probability subtracted from 1 to the variance is the coefficient of kurtosis of binomial probability distribution. Use the calculator & formulas for quick calculations or reference to find P(x), μ, σ2, coefficient of skewness or coefficient of kurtosis.
Formulas to Estimate P(x), μ, σ2, Skewness & Kurtosis
The below formulas are the mathematical representation to find combinations, probability of x number of successes P(x), mean (μ), variance (σ2), standard deviation (σ), coefficient of skewness & coeeficient of kurtosis from the binomial distribution having n number of finite trials or experiments. By using these formulas, users may get to know what are all the input parameters are being used in Binomial distribution formulas to perform such calculations manually. Users may use this Binomial calculator to verify the answers of such calculations or generate the complete worksheet for the corresponding input values.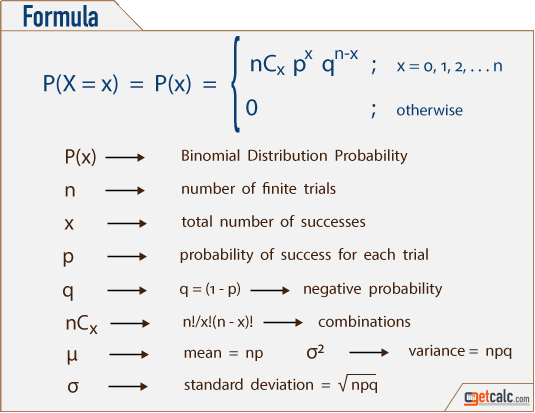
Example Problems with Solutions
The below are some of example problems with solutions generated by this calculator to help grade school students to solve similar Binomial probability worksheet problems effectively by just changing the input values.

